Equation of a Line
The equation of a line is a linear relation between two variables x and y, which is satisfied by the coordinates of each & every point on the line and not by any other point.
There are various forms of Equation of a line.
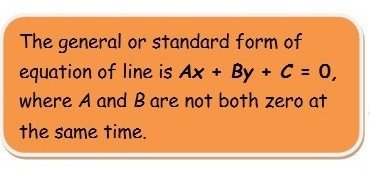
Example:
- 2x + 3y + 7 = 0 which can also be written as 2x + 3y = (-7).
- 4x + 3 = 7 where B = 0.
- 3y + 2 = 5 where A = 0.

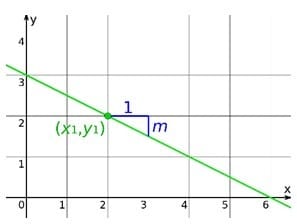
Point Slope Form
Equation of a line with slope or gradient m and passing through the point (,
) is given by
( –
) =
(
–
)
Example:
- Equation of line passing from origin (0, 0) and slope 1 is (y – 0) = (x – 0) or y = x.
- Equation of line passing from (1, 3) and slope 4 is (y – 3) = 4(x – 1) or y – 3 = 4x – 4
Hence the line is given by the equation 4x – y – 1 = 0.
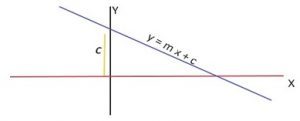
Slope Intercept form
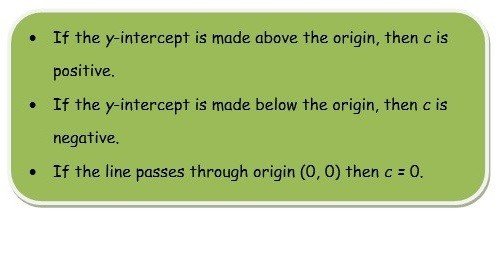
The length of the intercept made by of a line on the Y-axis is called its y-intercept.
Equation of a line with slope or gradient m and making an y intercept of c units is given
by (y – c) = m ( x – 0 )
i.e y=mx + c
Example:
- Equation of line with slope, m =
& y-intercept c = 7 is y =
x + 7.
- Equation of line with slope, m = 11 & y-intercept c = -2 is y = 11x – 2.

- Write the equation of the line 3x = 4y + 7 in general form.
- Convert the equation 3x = 4y + 2 in slope intercept form.
- Find the equation of the line passing from origin (0, 0) and slope 4.
- Find the equation of the line passing from origin (1, 5) and slope -7.
- Find the equation of the line with slope 2 & y-intercept 11.
- Find the equation of the line with slope
& y-intercept -5.
Answer key
- 3x – 4y – 7 = 0.
- Y =
x –
- y = 4x
- 7x + y – 12 = 0.
- y = 2x + 11
- y =
x – 5 or 3x – 7y – 35=0.
IN THE NEWS

Our mission is to provide high quality online tutoring services, using state of the art Internet technology, to school students worldwide.
Online test prep and practice
SCAT
SSAT
ISEE
PSAT
SAT
ACT
AP Exam
Science Tutoring
Physics Tutoring
Chemistry Tutoring
Biology Tutoring
Math Tutoring
Pre-Algebra Tutoring
Algebra Tutoring
Pre Calculus Tutoring
Calculus Tutoring
Geometry Tutoring
Trigonometry Tutoring
Statistics Tutoring
Quick links
Free Worksheets
Fact sheet
Sales Partner Opportunities
Parents
Passive Fundraising
Virtual Fundraising
Our Expert Tutors
Safe and Secure Tutoring
Interactive Online Tutoring
After School Tutoring
Elementary School Tutoring
Middle School Tutoring
High School Tutoring
Home Work Help
Math Tutors New York City
Press
©2022 eTutorWorld Terms of use Privacy Policy Site by Little Red Bird
©2022 eTutorWorld
Terms of use
Privacy Policy
Site by Little Red Bird






